Solution pour le challenge n° 85

Solution pour le challenge 85

Considérons une bijection continue $f:\mathbb{R}\rightarrow\mathbb{R}$ admettant $+\infty$ pour limite en $+\infty$ et montrons que sa réciproque possède la même propriété.

Comme $f$ est continue (sur un intervalle) et injective, elle est strictement monotone. Et vue sa limite en $+\infty,$ elle est croissante. Il s’ensuit que pour tout $A\in\mathbb{R}$ :

$x\geqslant f\left(A\right)\Rightarrow f^{-1}\left(x\right)\geqslant A$

et ceci prouve que :

$\fcolorbox{black}{myBlue}{$\displaystyle{\lim_{x\rightarrow+\infty}f^{-1}\left(x\right)=+\infty}$}$

Maintenant, montrons que cette conclusion n’est pas maintenue sans l’hypothèse de continuité.

Commençons par construire une bijection $g:\mathbb{Z}\rightarrow\mathbb{Z}$ vérifiant :

$\lim_{n\rightarrow+\infty}g\left(n\right)=+\infty$

et telle que $g^{-1}$ n’admette pas $+\infty$ pour limite en $+\infty.$ Pour cela notons :

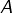$A$ l’ensemble des entiers strictement négatifs
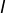$I$ l’ensemble des entiers naturels impairs
$B=A\cup I$

Soit $\varphi:A\rightarrow B$ une bijection ( $A$ et $B$ sont équipotents en tant que parties infinies de $\mathbb{N}).$ Considérons l’application :

$g:\mathbb{Z}\rightarrow\mathbb{Z},\thinspace n\mapsto\left\{ \begin{array}{cc}2n & \text{si }n\in\mathbb{N}\\\\\varphi\left(n\right) & \text{si }n\in A\end{array}\right.$

Il est clair que $g$ est bijective et que $\lim_{n\rightarrow\infty}g\left(n\right)=+\infty.$ Mais, pour tout $p\in\mathbb{N}$ :

$g^{-1}\left(2p+1\right)<0$

donc $g$ n’admet certainement pas $+\infty$ pour limite en $+\infty.$ Considérons alors :

$\boxed{f:\mathbb{R\rightarrow\mathbb{R}},\thinspace x\mapsto\left\{ \begin{array}{cc}g\left(x\right) & \text{si }x\in\mathbb{Z}\\\\x & \text{sinon}\end{array}\right.}$

On constate que $f$ est bijective et que, pour tout $x\geqslant0$ :

$f\left(x\right)\geqslant x$

et donc, par comparaison :

$\lim_{x\rightarrow+\infty}f\left(x\right)=+\infty$

Mais $f^{-1}$ ne tend pas vers $+\infty$ en $+\infty,$ puisque la suite $\left(f^{-1}\left(2p+1\right)\right)_{p\in\mathbb{N}}$ ne diverge pas vers $+\infty.$

Pour consulter l’énoncé, c’est ici

Partager cet article

Laisser un commentaire Annuler la réponse